
www. e l e c t r i c a l c o nn e c t i o n . c om . a u
4 5
with
Chris Halliday
the reactance will have little effect on the
total impedance and so can often be ignored.
Ohm’s law is used for this method as
follows: Vd = I x Zc.
Where:
>
Vd = voltage drop in volts
>
I = circuit current in amps
>
Zc = impedance of the circuit
Most sparkies will be more familiar with
the second method: Vd = (L x I x Vc)/1000.
Where:
>
Vd = voltage drop in volts
>
L = route length of the circuit in metres
>
I = circuit current in amps
>
Vc = cable voltage drop per ampere-
metre length of circuit in millivolts per
ampere-metre (mV/Am)
The value of Vc is provided by Tables 40-
51 of AS/NZS 3008.1.1.
The formula can also be rearranged to
calculate Vc then look up a cable with a
mV/Am value less than the value specified
(not equal to, as there is no built-in margin
for this simplified method).
Tables 40-51 are for three-phase circuits.
Single-phase values of Vc require the
three-phase values to be multiplied by
1.155. Don’t get caught by this little trap for
young players.
The value of Vc is based on the maximum
conductor temperature permitted by the
cable insulation material. Allowance can be
made if the cable operating temperature
is considerably less than the maximum
(refer Clause 4.4 of AS/NZS 3008.1.1) and for
differences in power factor.
The values of voltage drop calculated
can be converted to a percentage by
multiplying by 100 then dividing by 230
(for a 230V system).
The Wiring Rules has a simplified version
of the latter method at Table C7. You simply
need to multiply the length in metres by
the current in amps and divide by the
allowable voltage drop percentage, then
find the size of cable with a value more
than that provided by the Table C7.
EXAMPLES
A sparkie wants to connect a normal 16A
single-phase power circuit using standard
power cable – 2.5mm² twin and earth, PVC
and PVC thermoplastic sheathed.
Voltage drop in the consumer mains and
sub-mains has been calculated, and 3% is left
of the allowable 5% for the circuit. The final
socket outlet has a route length of 40m.
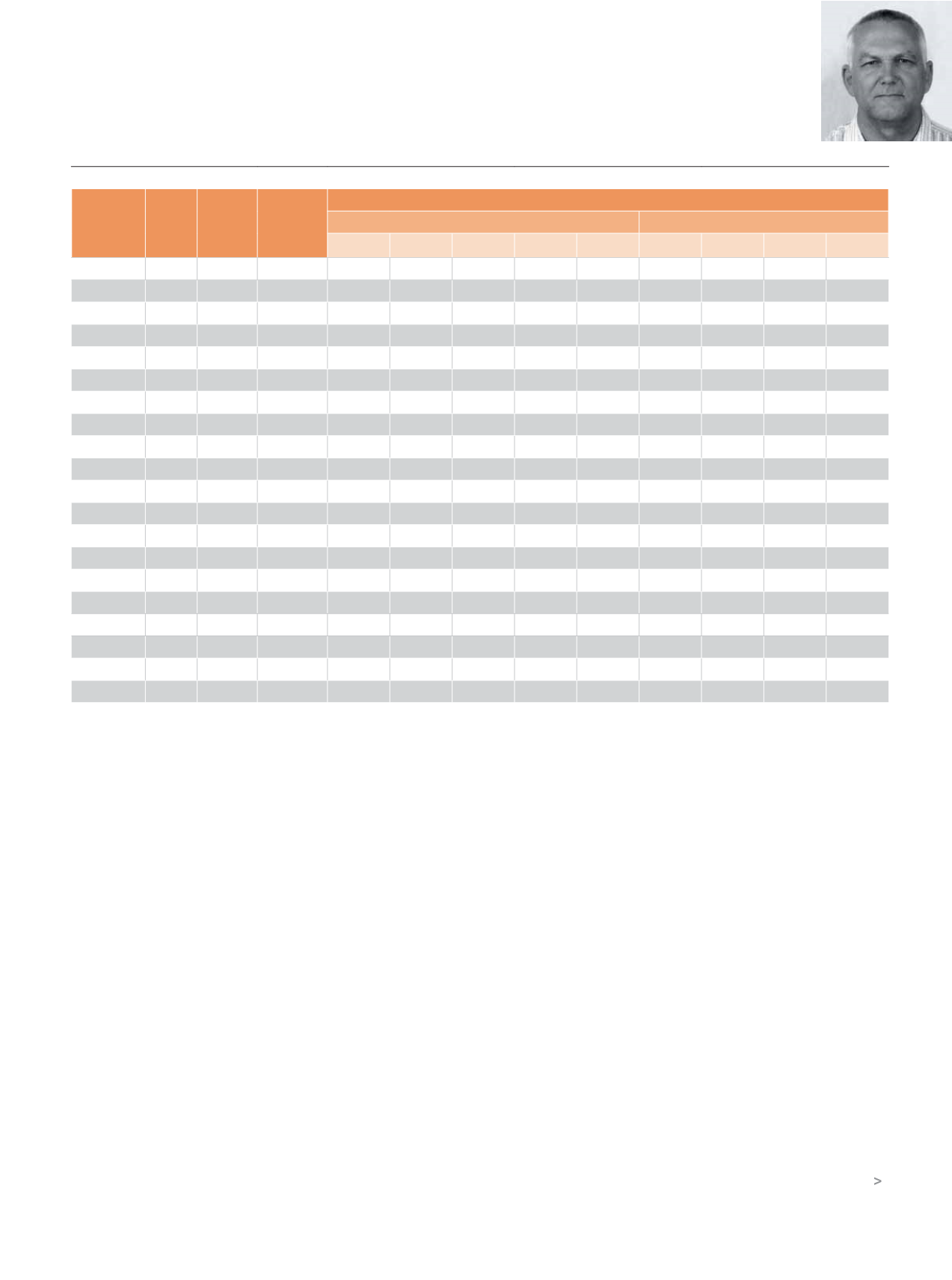
Table 1 - Voltage Drop Requirements Compared to Loop Impedance - Maximum Route Lengths
Active(s)
&/or
Neutral
(mm²)
Earth
(mm²)
No. of
Phases
Protective
device
rating (A)
MAXIMUM ROUTE LENGTH
Voltage Drop (%)
Loop Impedance (Table B1)
2% 3% 4% 5% 6% Type B Type C Type D Fuses
1
1
1
6
14.8
22.3
29.7
37.1
44.5
170
91
55
204
1
1
1
10
8.9
13.4
17.8
22.3
26.7
102
55
33
114
1.5
1.5
1
10
13.9
20.9
27.9
34.8
41.8
153
82
49
170
1.5
1.5
1
16
8.7
13.1
17.4
21.8
26.1
96
51
31
82
2.5
2.5
1
16
16.0
23.9
31.9
39.9
47.9
160
85
51
136
2.5
2.5
1
20
12.8
19.1
25.5
31.9
38.3
128
68
41
93
4
2.5
1
25
16.4
24.6
32.8
41.0
49.2
126
67
40
90
4
2.5
1
32
12.8
19.2
25.6
32.0
38.5
98
52
31
70
6
2.5
1
40
15.3
23.0
30.7
38.4
46.0
90
48
29
60
10
4
1
50
20.6
31.0
41.3
51.6
61.9
117
62
37
73
16
6
1
63
26.0
39.0
52.0
65.0
78.0
142
76
45
85
16
6
1
80
20.5
30.7
41.0
51.2
61.5
112
59
36
59
25
6
1
80
32.3
48.5
64.7
80.8
97.0
124
66
40
66
25
6
1
100
25.9
38.8
51.7
64.7
77.6
99
53
32
47
35
10
3
100
41.4
62.2
82.9 103.6 124.3
159
85
51
75
35
10
3
125
33.2
49.7
66.3
82.9
99.5
127
68
41
58
50
16
3
125
44.4
66.6
88.8 111.0 133.2
198
106
63
90
50
16
3
160
34.7
52.0
69.4
86.7 104.0
155
83
50
71
70
25
3
160
49.3
74.0
98.6 123.3 147.9
235
126
75
108
70
25
3
200
39.5
59.2
78.9
98.6 118.4
188
100
60
84
Notes:
1. Voltage drop values are calculated using 75°C values from Table 42 of AS/NZS3008.1.1
2. Double the route length where the load is distributed over whole length of the circuit such as a lighting or socket outlet circuit.
3. For smaller three phase circuits multiply the route length of any single phase circuits above by 1.155.
















